Chris (@chris2306) got asked how fast you would need to be going to complete a loop the loop. This is what we got.
We are going to find the minimum speed you require to complete the loop, we’ll do this via an energy argument. For ease, we’ll ignore friction! First we need to find the minimum speed required at the top of the loop.
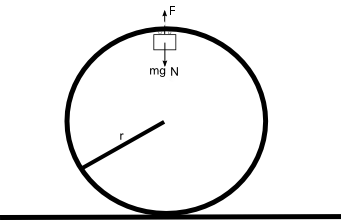
To get the minimum required speed to make the loop the loop, at the top of the loop we require the normal force (\(N\)) to be 0. Equating the forces at the top of the loop we have the weight of the car (\(mg\)) plus the normal force (\(N\)) equal to the centrifugal force (\(F\)), which is given by \(F=\frac{mv^2}{r}\) where \(r\) is the radius of the circle. Thus,
\begin{eqnarray*}
\frac{mv_t^2}{r} &=& N+mg, \\
\frac{v_t^2}{r} &=& g,\\
v_t^2 &=& gr,\\
v_t &=& \sqrt{gr}.
\end{eqnarray*}
Giving us a minimum velocity at the top of the loop, \(v_t\). We now proceed with the energy argument.
The total energy at the top of the loop is equal to the potential energy at the top plus the kinetic energy at the top, respectively these are: \[PE_t = mgh = 2mgr,\] \[KE_t = \frac{1}{2}mv_t^2 = \frac{1}{2}mgr.\] Thus the total energy at the top is:
\begin{eqnarray*}
E_t &=& 2mgr+\frac{1}{2}mgr,
&=& \frac{5}{2}mgr.
\end{eqnarray*}
We now find the total energy at the bottom, since there is no potential energy, this is simply the kinetic energy, \[E_b = \frac{1}{2}mv_b^2.\] Where \(v_b\) is the speed we are looking for. With our assumptions, the energy at the top (\(E_t\)) equals the energy at the bottom (\(E_b\)) giving
\begin{eqnarray*}
E_b &=& E_t,\\
\frac{1}{2}mv_b^2 &=& \frac{5}{2}mgr,\\
mv_b^2 &=& 5mgr,\\
v_b^2 &=& 5gr,\\
v_b &=& \sqrt{5gr}.
\end{eqnarray*}
Thus we have found the speed required to complete a loop the loop of radius \(r\). For example, if the loop had a 4 metre diameter (2 metre radius) then the velocity required to complete the loop would be \(v = \sqrt{5\times g\times 2}=\sqrt{10g}\approx 9.9\).
For convience we can approximate \(\sqrt{5gr}\) as \[\sqrt{5\times 9.81\times r}=\sqrt{49.05r}\approx 7\sqrt{r}.\]
—
Many thanks to Mike Barr for pointing out that the explanation from where \(v_t = \sqrt{gr}\) came from was incorrect. This has now been corrected.
“For ease, we’ll ignore friction!” is my favorite bit.
what if there is friction befor entering th loop?
thanks
In that case the efficiency is reduced. So….. If 15% of the energy is lost before entering the loop, then 0.85mgh=1/2 mv^2, doing the maths gets you h=5r/2*x% as a general equation.
Sir,
If the question is what is minimum velocity with which a body of mass “M” must enter a vertical loop of radius R, so that it can complete the loop.
what should be the answer: underfoot gR or underoot 5gR
Underoot 5gR, since it’s the initial velocity, or the minimum velocity with which a body has to enter a loop (Vb).
Underoot gR is the minimum velocity it needs when the body is at the top for it not to fall.
Maybe it’s a bit of a late answer, but I hope it helps
I am using this page to see if my HS physics students can catch the mistakes. First, at the top the net (or resistance force) should not be zero. The Centripetal force (labeled N here) is not up but down. The Normal force should be zero at the minimum possible velocity. So, the net force and only thing keeping the object in a circle will be mg. So, the equation is correct but the explanation is just wrong.
Thanks for your comment Mike. Yes the mistake came from my mixing up the words centripetal and centrifugal! Also by using N to label the centripetal force is clearly confusing when that is usually reserved for the normal force. Fortunately the equations still worked out, but you’re right the explanation was incorrect. I’ve now updated the post. Thanks again. Sean.
can you explain to me because i can’t understand this point
why the reaction (N) is downwards?
The Normal force acts perpendicular to the surface, i.e. the arrow points away from the floor. There is some more details on this Wikipedia page.
thanks for illustration
A car is coasting (the car is in neutral with the engine off) but moving very quickly. The car has a mass of 2036 kg. The car goes through a loop as shown in the picture. How much kinetic energy will be lost when the car is at the top of the loop if the radius (r) is 28 m?
Note that you should take inertia in account when this is a rolling ball. Ignoring friction I received: v =sqrt((27/7)g(R-r)) as the minimal velocity at loop radius R and ball radius r.
I thought centripetal (centrifugal?) Force always acts towards the centre if the circle, thus creating the circular motion, and the reaction forces are felt ‘outwards’. If this is the case why have you labelled the centripetal force F as going ‘out’ and normal force going towards the centre?
Hi Fintan, centripetal acts inwards (like you rightly say), centrifugal acts outwards. Whilst centrifugal is a “pseudo” force, it works fine for this problem (I really like this xkcd about the two forces). Thanks, Sean.
If the particle is located where in the above diagram the radius line touches to the surface then what will be the minimum velocity to complete the loop
What would happen if you created too much energy before entering the loop?
If centrifugal force is an imaginary force, When and How can you use centrifugal force to solve problems?
What happens when the string has mass ?
The only force acting on the (non-rotating) body at the top of the loop is that required to keep it in circular motion with a radius ‘r’, and this force is provided by its weight. The centripetal acceleration is vw (where ‘v’ is linear velocity and ‘w’ is angular velocity. Since v = rw, or w = v/r, it follows that the centripetal acceleration is also given by v^2/r. So the centripetal force is given by the usual F = ma, which in this case is mv^2/r, and this is being provided by its weight, given by mg. So, mg = mv^2/r and so v = sqr.root(gr). Done.
So in normal
Folk talk how fast should you be driving in your car MPH? please 🙂 and thank you. I was lost after centrifugal force lol
Firstly, I would like to thank Mr Elveridge for making this post. I remember in my youth that we had to create a straw rollercoaster for a ping-pong ball. I found the same problem and wrote to my friend a letter. He later became the physicist John Parkes. He would have been greatly helpful in my time. ANyway GREAT job!